Electronics - PT100 temperature sensor (Resistor networks)

Created; 05/04/2018, Update; 30/06/2025, 29/06/2025

Temperature measurement, this is also an addendum to the blog page: Electronics - Robust electronics design example - outdoor wireless environmental monitor. 

PT100 Temperature sensor.

Using a PT100 or other resistor temperature sensor is probably a better option than using a semiconductor sensor, where electrical interference levels are higher.  Another option is a combination of thermistors. 

 

RTD such as PT100 or PT1000 are fairly linear over at least -50 °C to 200'C or much wider, there is a slight curve.  But one of these may have been selected if the circuit and PCB design could not have been fitted in the free version of the CAD chosen.

• NTC Thermistors can be programmed with a series resistor to be roughly linear over a 40 °C temperature range.
• Two thermistor-type temperature sensors are roughly linear over a 100 °C temperature range but have a wobbly error graph. 

• Semiconductor-type temperature sensors, such as IC types such as AD590, work well, and would benefit from a 100nF capacitor for reducing electrical interference.  Works over and is linear over -55 to 150 °C. 

Component selection; Sensors;

PT100 temperature sensors on 4-wire cable + instrumentation amplifier.  The wires, if kept (e.g. twisted) together, should not need more screening; the filtering should be good enough to deal with cabling acting as an aerial (I have moderated my earlier caution as a consequence of more recent experience).  The temperature input/output series resistors and capacitors should serve this function well.

Circuit 1 below is a two-channel temperature sensor using a chain comprising two reference resistors and two PT100 temperature sensors.  The circuit was modified to include only one temperature channel and therefore works with a single reference resistor.  Later, I explore chopper stabilisation.  The analogue circuitry could have 1nF capacitors at the boundary of the circuit board the analogue multiplexer will be changed from the circuit below to a dual four channel multiplexer which also provides some ESD protection other channels for calibration 100nF capacitors to the input of an instrumentation amplifier an output resistor in series with the capacitor and an analogue input to of the Xbee module.

1. Draft design for discussion;

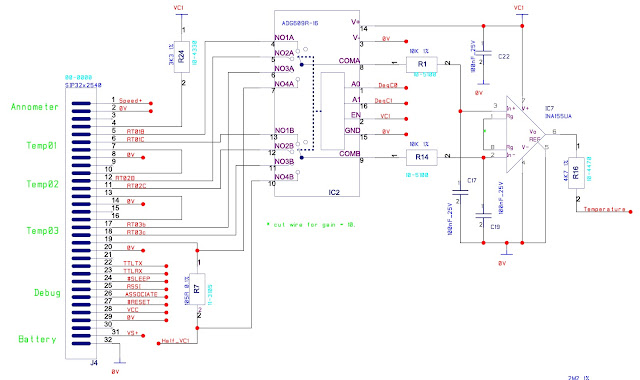
AL-0016-01B The temperature measurement section of the circuit.  Created with CADSTAR 16.0 Express.  This first draft of the circuit won't work; the instrumentation amplifiers' reference input should be at least 0.8V above 0V or Vdd-0.8V.

Resistance measurement is scaled by the resistance of the PT100 + R7 + R10.  The calculation is circular, where the answer is required to do the calculation.  If a typical temperature value of, say, 105 ohms is used, then an approximation will be found.  In a continually running system, the next time the temperature is measured using the previous approximation of the measurement will be accurate. 

 

 

R10 could be another PT100 temperature sensor; in that case, the accuracy will be limited by the instrument amplifier's gain error of 1%:

 

That is; 1% of PT100 maximum 1.25R = 125R/100  [65'C maximum]

  Resistance to temperature 3.3'C  = 1.25R / 0.385     [ohms per 1'C]

  Plus the offset error. 

 

This is not good enough, so the options are:  

1. Change the Instrumentation amplifier to a INA155UA; this will work with 0V input at its Ref input, so the output will have a bigger voltage swing to improve the A/D resolution. In addition, its gain accuracy is better at 0.1%, but its offset is bigger. This solution should work if a lower x10 gain is selected and a higher resistor chain current, so that larger signals are used. In this case R10 can be replaced with a second PT100 temperature probe. 

2. Change R10 to a precision resistor, and use it as a single point of scale factor calibration.  R7 becomes not part of the calibration system and a general-purpose resistor would suffice. 

I did not understand Figure 6 in the AD8293 data sheet.  It turns out that the pink area represents the lowest supply voltage operation at 2.7V, and the blue area, including the outer pink border, is for 5V operation.   Here is my question on what Figure 6 means answered

Another option is to reduce the gain of the instrument amplifier, thereby increasing the noise immunity,  by placing a resistor across the feedback filter pins.  This retains the bandwidth limiting with the capacitor, but the gain accuracy is then reduced by up to 20%.  This option is not worth pursuing further. 

-------------------------------------------------------------

Replacing the instrumentation amplifier and multiplexer with a Cirrus Logic delta-sigma analogue-to-digital converter would make a very accurate temperature measurement system.  This particular maker's products are easy to use and are known for being accurate, such as in the audio and instrumentation industries.  On the other hand, the temperature accuracy could be very high, but there is some extra software work to do, whereas the first solution largely uses already included firmware in the wireless module. 

CS5531, CS5532, CS5533 or CS5534, for example, is easy to use, more accurate and just requires the series resistor chain.  It includes up to a 4-channel multiplexer fed to a single A/D.  You don't need to use the Programmable Gain Amplifier, which, in any case, would have required a calibration cycle.  So one of these could be used with one or four temperature probes and one precision reference resistor connected to the reference input, thereby also making the measurement immune to low-frequency common-mode electrical noise.  The calibration selection of the IC serves no purpose; the IC is accurate from turn-on. 

The opinion of sales representatives and other engineers is that the significant patents from about 1990 expired long ago, and all manufacturers of Delta-Sigma or Sigma-Delta type A/D converters should now be equally easy to use and similarly without the 100uV noise spikes that some of them suffer with. 

I have used this with 0.01% accurate resistors as part of the calibration system, which gives a potential temperature accuracy of 0.05 °C, but it is not possible to trace temperature calibration to this accuracy.  Each PT100 was soldered to a PCB along with connector, an I2C bus EEPROM which held a calibration constant for that temperature probe.  It is often the case that instruments resolve confidently to a much higher accuracy than the calibration can be traced by a national standard to. 

In any case, the instrumentation amplifier solutions below, connected to an embedded microcontroller A/D, work well. 

--------------------------------

 

Component selection - Instrument Amplifiers;  

                    

1. AD8293G80 Ref input works best at mid power supply.

2. INA155UA Ref input works at mid supply, or if the inputs are near one power supply, the Ref input should be closer to that power rail.  That gives this amplifier a bigger output range in these circuits, which is beneficial, spreading the signal over more of the wireless module's A/D input range. 

Both amplifiers give better results when multiplexed and chopper-stabilised.  It turns out that chopper stabilisation is not required, but multiplexing with a precision resistor in the resistor chain as one of the channels as part of a self-calibration system is a good strategy.

1. The first amplifier is the cheapest and the gain accuracy is poorer, but it is chopper stabilised and the offset error is better.  The outputs and the inputs in any case should not be operating too near their voltage range limits to avoid inaccuracy due to noise and interference.

2. The second amplifier is the fastest.  Can be multiplexed and chopper stabilised at some kHz and thereby move the frequency away from noisy low-frequency noise. 

• If the Ref input and the resistor chain are switched, 0V and Vdd give two sample points per cycle of the A to D, so there should be at least 50% better accuracy by averaging.  Any contact junction voltage offsets are eliminated, but switching noise and cross-coupling of synchronous signals would be introduced unless good care with screening and PCB layout is taken.  See circuit 3.
  

Spreadsheet of temperature options and design arithmetic -  This blog is not a step-by-step log of a design process, but is more like a discussion that progresses through options. 

The accuracy is better than 1 °C using most options, but it later turns out that better accuracy is possible with any option. 

 

Accuracy due to the A/D 

10-bit accurate 12-bit resolution.  With some increase in resolution and accuracy by applying noise reduction using a mode filter, averaging and but it turns out that chopper stabilisation does not improve the accuracy of the system. 

 
A temperature accuracy of, say, 2 °C would have been good enough to proceed with the design. 

Self-heating of the Pt100

PT100 raw element self-heating 0.5'C/mW in air. RS ceramic types 1.2mm x 1.6mm or larger.  Self-heating could be a lot more with a simple covering, such as a heat-shrink sleeve. 

Self-heating of the temperature sensor is small or very small in most circuit options.

I have in the past soldered 4 wires and potted a PT100 or PT1000 temperature sensor into a blind tube, such as a bolt with a deep head milled out.  That was using a thermally conductive, electrically insulating epoxy resin. 

Temperature range -20 °C to 50'C we allow for about 100 °C, say 139 ohms.  That is about 80% of the power supply noise margin. 

Mid temperature 13 °C, 105 ohms, 0.1% calibrator resistor. 

Spreadsheet of parts selection is lost, but in conclusion, ADG609R-16 or MAX4559CSE were chosen. 

Not all the accuracy issues have been considered; for example, linearity error has not been considered.  Provided you give yourself time, the significant tolerances will have been considered by the end of the design cycle.  The noise immunity part of the design is an art that comes with an understanding of RF design, care and experience. 

------------------------------------

 

2. Circuit corrections and improvements;

 

This is a near-ideally screened circuit because it has the best placed filtering at the i/o boundary.  But it uses too many components in this particular case. 

 

Revised 3-channel temperature circuit AL-0016-02c. The filter is similar to the recommended 1n3F plus 39nF at the output, which won't change the settling time much. Ref input is 1/3rd V+ but is 1% tolerance, which may compromise the temperature accuracy. Placeholders for filter capacitors are shown as missing components; if the placeholders were filled, the multiplexing speed would be compromised depending on the size of the capacitors.

This level of input filtering should work well with long unscreened cables in a moderate electromagnetic field environment.  I usually filter to this degree for short cable run applications, and nothing adverse has arisen from doing that.  But if this degree of filtering were required, then many more measures would be required, such as screened cables and using a metal enclosure.  

 

A circuit error has been corrected by adding a 1/3rd V+ rail for the Ref input.  That is 1% accurate (11mV), which may introduce a significant error to the measurement.  Approximate accuracy; 

• The output voltage range is 1/3rd to 3/3rds Vc.  2.2V.
• Maximum ohms (range 144R) from the spreadsheet.
  
• Sensitivity  ~6mV = 0.385R * 2.2V/144R, per 1'C
• Therefore, the accuracy of ~11mV corresponds to 2'C accuracy.
• But only part of the range is used; ~0.5'C = 2'C * 15 ohms / 144 ohms with single-scaling-point calibration system. 

This approximation is wrong, but it is good enough to show that adding a 1/3, 1% supply voltage divider to proceed.  The maths can now be tidied up, checked and entered into the spreadsheet later; meanwhile, I pursue other options. 

The reason this circuit was not used is that there were too many components for the CAD tool when other parts of the circuit were developed more, plus the amplifier IC footprint is small and a risk for hand soldering, though I have hand-soldered this density footprint before. 

Examples of EMC measures

Temperature measurement function software workload

• Cycle through all four channels.

• Allow a settling time, then take three readings for each channel so that mode averaging can be applied, that is, drop the highest and the lowest readings.

• Optionally, the mode-averaged measurements can be summed (which amounts to averaging) to further reduce the noise.  The square root of the number of samples, if there is noise and the noise distribution is normal and of a reasonable amplitude at best, can increase in resolution 10X for 100 readings.
• Note that the successive approximation A/D on the wireless module must have a well-filtered input, or else high-frequency noise will introduce big errors. 
• For each channel, do the sum; Temperature n = (105 * Channel n / Channel 4 {calibrator} - 100) * 0.385. 

The instrumentation amplifier includes chopper stabilisation, but this is not a chopper-stabilised system.  The circuits below include full system chopper stabilisation.   

From the spreadsheet, the accuracy is ~0.5'C.  The scale factor correction is done at one point about 13 °C, and the zero by the intrinsic accuracy of the instrument amplifier, Ios Vos.  The offset errors are further reduced because the total resistor chain resistance is high compared to the working resistance range. 

----------------------------------------------------------------------

3. Chopper stabilised temperature measurement

Evaluation of full chopper stabilisation, including the resistor chain and, in this case, Ref input switching.  Although it turns out that the required accuracy has been achieved without chopper stabilisation.

 

The circuit must be well screened with a 0V plane on the PCB.  The result can be awful to amazingly good.  The link to a light and photo diode system, worked in a system which worked very well even when exposed to ambient room lighting greater than the signal of interest;  electronics---exception---synchronous-rectifier

AL-0016-03D Evaluate IN155UA further, but with chopper stabilisation, plus Ref input switching so that the output signal amplitude is maximised and uses two parts of the analogue to digital converter's range.  The input filtering has been reduced and moved, but it is as good a filter, and the mux includes ESD protection for much higher noise levels.  The accuracy may be compromised by variable contact and cable resistance.  This circuit has an extra, fourth temperature channel. 

 

The instrument amplifier chosen has a very small footprint.  Even though I have hand-soldered this package but it would be better to use INA155 with the larger SO-8 footprint.  Using two reference resistors was another option evaluated, one of those being 10R 1%  (as a near-zero reference point) and the other 130R 0.1% (span reference point).  This is approximately the solution I first used before. 

• INA155 settles in 15uS to 0.01%. The cycle is the four mux channels twice is 8. 
  

• Time constant of the final RC formed by the analogue mux and 10nF.  10uS = 1K * 10nF, and a rule of thumb given at college was that the circuit will settle in 5 time constants. 
• If the voltage steps are, say, 10x bigger than the temperature signal, the time constant is 10uS + 15uS, and we need 10 time constants to get to 0.01%.  Use 250uS settling and 8 steps per cycle is 2mS. 
• That is, 2mS is a chopper rate of 500Hz maximum. 
  
• AD8293 G80 with the recommended compensation 1.3nF and output filter 39nF, settling time to 0.01% is 2.4mS. 
• 8 cycles is a maximum chop rate of 52Hz. 
• Ref input can not be chopped rail to rail, but only about +-0.5V (2.7V Vdd minimum) or held at a minimum supply. 
  

The rule of thumb, 5 time constants that a circuit will take to settle, was given to me at college, but checking the figure, e^5 is approximately 150.  So the rule of 5 time constants works to 0.67%.  But the voltage swing is large, 3.3V peak to peak, and the resolution is 0.01% [0.385 / 3,800]  for 1'C accuracy.  10 time constants will settle to about 0.005%. 

 

Chopping frequency;

The measurement cycle has 8 steps, four channels twice.  

• Ideally, a high frequency should be used; 500Hz is possible.
• A sub-multiple of 50 and 60Hz is 10Hz. 

• The last option is to chop at the main frequency of the country, 50Hz or 60Hz.  In a vintage instrument (Polarimeter P70-4) that used mains frequency, this works very well until the synchronous motor's bearing wears, the motor slows slightly, resulting in a low-frequency beat with mains frequency, and the user sees a flickering

  reading

  . 

 

Temperature measurement function software workload

• The software should cycle through all channels, switch polarity and cycle through all channels again.  A variable settling time is required depending on the voltage change between each step of the cycle, or a larger settling time be used. 

• For each channel switch, allow a settling time, then take three readings so that mode averaging can be applied, that is, drop the highest and the lowest readings. 

• Each channel is summed with the polarity switched readings subtracted from the positive polarity readings, so that the offset error is eliminated. 

• A number of cycles should be summed for better noise reduction and resolution improvement. 

If software were running within the XBee wireless module, then local chopper stabilisation could be run with better timing than if run over the wireless connection.  In that case, running chopper stabilisation is an unnecessary complication, and a higher frequency chopping is hardly possible.

--------------------------------------

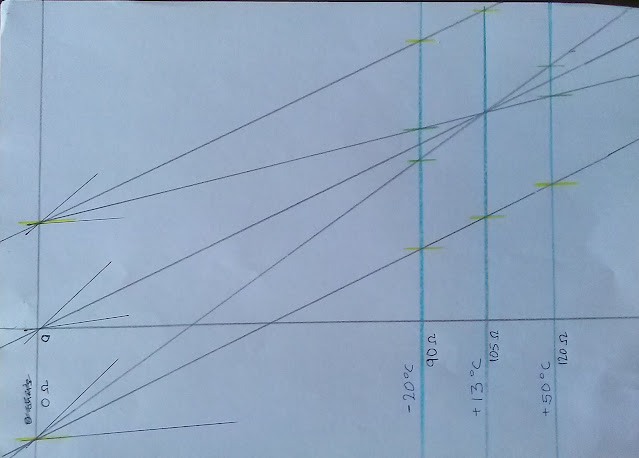
The temperature range uses a small part of the resistance range and an even smaller part of the total resistance of the resistance chain.  I could not make up my mind how much this using a small part of the total resistance improved the accuracy, so I drew a picture.  This made what would occur and what would not happen clearer, and I drew many versions of this graph before this one below. 

 

It turns out that the total resistance chain is only relevant to how big the signal is to the amplifier, and the smaller the signal, and higher the gain, the system will have a poorer noise margin.  On the other hand, the higher gain AD8293 has more low-pass filtering integrated.

 

The three diagonal Pencil lines are the ideal and offset error lines due to amplifier offset.

The Blue Biro lines that peter out are the amplifier gain and offset errors.  

• Blue Biro lines would be the significant errors if Circuit 1 were developed.  From the spreadsheet INA155 with the gain set to 10 option and the measurement chain resistance of ~720 ohms is promising.  In that case R10 becomes a second temperature channel. 

• Blue Biro lines emanating from the origin 0 are relevant to the chopper stabilised Circuit 3 in that case, though the resistance and gain accuracy using INA155 is much better and the required accuracy would be met. 

• Blue Biro lines are not relevant errors in Circuits 2 and 4 due to the single scaling point calibration system. 

The Pencil lines intersecting with the 105-ohm calibration point show the accuracy improvement brought about by this single scaling point correction strategy (circuits 2 and 4).

• The Yellow pencil marks show the offset error without the calibration.  This would have been the biggest error in circuit 3 that uses INA155 with a gain of 50.  Improved using the gain option of 10. 

• The Green pencil marks are the new improved by calibrated errors because of the smaller working resistance range -20 to +50 °C (92R to 120R).
  

The scale of this picture is exaggerated to demonstrate the point.  The errors are too small to see when scaled the same as the temperature range and the other factors. 

In conclusion, a single mid-range scaling point self-calibration system will work well.  The amplifier gain or offset errors are not significant; either amplifier AD8293G80 or INA155UA would work well. 

---------------------------------------

 

4. Less input filtered INA155UA instrumentation amplifier solution;

This circuit has less input filtering than the ideal circuit (-02?).  In mitigation, the input is centred mid supply so that noise is less likely to be big enough to cause the mux protection diodes to conduct distort and so introduce error. 

 AL-0016-04E CADSTAR 16, Less interference filtered INA155, but to mitigate the input is centred at mid supply. 

The final circuit uses the INA155UA, but is set at its lowest gain and with greater low-frequency filtering for better interference immunity.  Therefore, the input signals are bigger and the RTD current is higher, but even so, the RTD self-heating should be modest.  Consequently, the filtering has been increased so that the chop rate and multiplex rate are set lower, <=50Hz. 

Spreadsheet of temperature options and design arithmetic is now working well, and options can be added easily as long as the variants are placed horizontally and the outputs are placed in the same place (horizontally) with the different parameters stacked vertically.  If reorganised by cutting (not copying) and pasting, that tends to make the spreadsheet easier to develop as you work on it. 

 

The accuracy is similarly good with all circuits, but in this option, the amplifier gain is a little lower, and if the self-heating due to the temperature is unlikely to be an issue, the gain could be reduced.  In that case, the incalculable RFI factors would be less harmful to the measurement.  That is R24 becomes 300R, and the wire is cut between IC7 pins 1 and 8.

 

-------------------------------------------------------------------------------------

An engineer can apply worst-case design tolerance and, by using a statistical approach, ensure that most products, when assembled, will work.  That is close to how raw material and measured parameters come from nature and are first processed for use!  But supplied components are made and specified, precisely and consistently, with all components meeting their tolerance specifications.  This is how electronics have changed since 1980.

Solving complex resistor networks by trying standard value components in your equations and seeing.  But first, do some maths. I do not solve using Maxwell's circulating currents and Superposition, but rationalise to simpler Norton's current source or Thevenin's voltage source equivalents.  But to come back to the first point, a resistor network needs to be solved to use standard value resistors and satisfactorily meet the design tolerances.  That tolerance is met by summing squares (of the errors), and this is called least squares.  In a basic program written that loops until a good fit is found, some BASIC let you stop, edit it, then continue.  I have done this, and it is powerful, but it is rarely necessary because simple circuits are mostly adequate to get usable data into a microprocessor, and then the arithmetic is carried out in that microprocessor precisely. 

Summing up;

It is now possible to buy close-tolerance components cheaply, and these do not have a traditional normal tolerance distribution, but virtually all of them will work within tolerance.  That is modern design work is now simpler; the part manufacturer has taken most of the design work out of your job.  But the art of low susceptibility to electrical interference and understanding RF is important. 

The PCB is more developed, and some other aspects have become clearer while working on the circuit.  So the designer should go back repeatedly and improve the design until a satisfactory solution is found.  For example the temperature range could be increased and the accuracy reduced. 

Conclusion

Electronics now uses a microprocessor this has simplified electronics to mostly using two resistor potential dividers and solving them using Norton or Theveni's current source or voltage source and running through preferred many resistor values until you find a solution that works. 

Connected blog;  Electronics - Robust electronics theoretical design example

Please comment below;